힙(Heap)

---

힙(Heap)?

우선순위 큐 : FIFO인 큐와 달리 우선 순위가 높은 요소가 먼저 나가는 큐를 우선 순위 큐라고 한다.


우선순위 큐는 자료구조가 아닌 개념이고 힙과 우선 순위 큐는 같지 않다. 단순히 우선순위 큐를 구현하는 방법 중 하나가 힙을 이용하는 것 뿐이다.

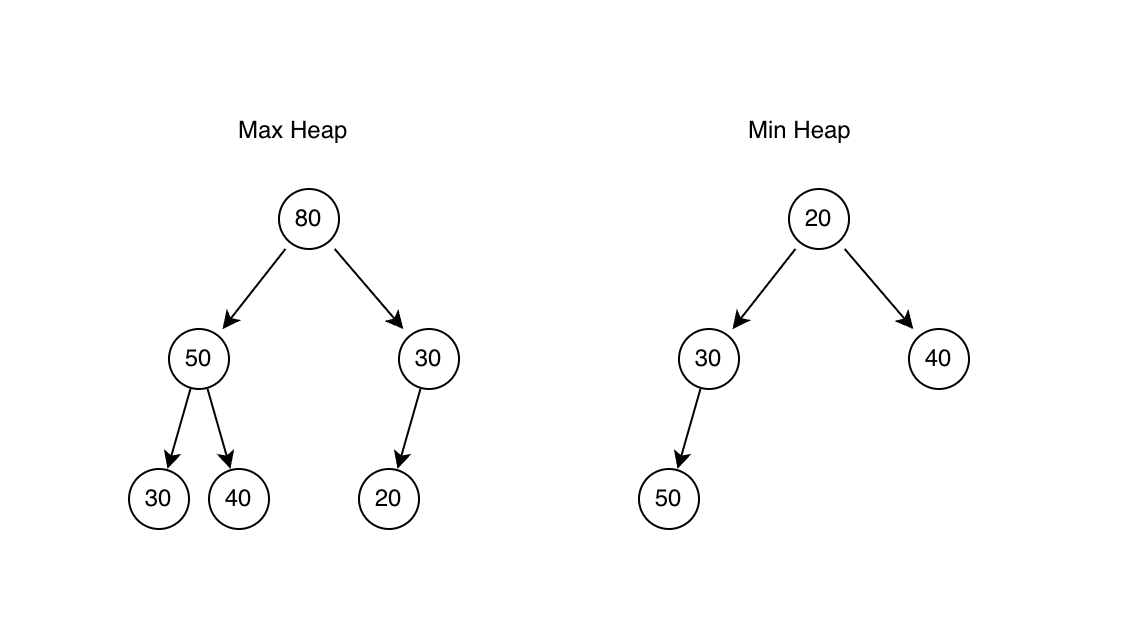
힙은 이진 트리 형태를 가지며 우선순위가 높은 요소가 먼저 나가기 위해 요소가 삽입, 삭제 될 때 바로 졍렬되는 특징이 있다.

힙 특징

• 우선순위가 높ㅇ느 요소가 먼저 나가는 특징을 가진다.
• 루트가 가장 큰 값이 되는 최대 힙(Max Heap)과 루트가 가장 작은 값이 되는 최소 힙(Min Heap)이 있다.
• 자바스크립트에서는 힙을 지원하지 않아 직접 구현해서 사용해야 한다.

힙의 추가와 삭제

힙의 요소 추가

• 요소가 추가될 때는 트리의 가장 마지막 정점에 위치한다.

• 추가 후 부모 정점보다 우선순위가 높다면 부모 정점과 순서를 바꾼다.

• 이 과정을 반복하면 결국 가장 우선순위가 높은 정점이 루트가 된다.

• 완전 이진 트리의 높이는 logN이기에 힙의 요소 추가 알고리즘은 O(logN)의 시간복잡도를 가진다.

  
  

  힙의 요소 삭제

• 요소 제거는 루트 정점만 가능하다.

• 루트 정점이 제거된 후 가장 마지막 정점이 루트에 위치한다.

• 루트 정점의 두 자식 정점 중 더 우선순위가 높은 정점과 바꾼다.

• 두 자식 정점이 우선순위가 더 낮을 때 까지 반복한다.

• 완전 이진 트리의 높이는 logN이기에 힙의 요소 제거 알고리즘은 O(logN)의 시간 복잡도를 가진다.

최대 힙 구현

class MaxHeap {
    constructor() {
        this.heap = [null];
    }

    getLeftChildIndex(index) {
        return index * 2;
    }

    getRightChildIndex(index) {
        return (index * 2) + 1;
    }

    getParentIndex(index) {
        return Math.floor(index / 2);
    }
    
    swap(index1, index2) {
        const temp = this.heap[index1];

        this.heap[index1] = this.heap[index2];
        this.heap[index2] = temp;
    }

    isEmpty() {
        return !this.heap[1] ? true : false;
    }

    push(value) {
        if(!value) {
            return;
        }
        this.heap.push(value);

        let currentIndex = this.heap.length - 1;
        let parentIndex = this.getParentIndex(currentIndex);
        
        while(parentIndex !== 0 && this.heap[parentIndex] < value) {
            this.swap(currentIndex, parentIndex);

            currentIndex = parentIndex;
            parentIndex = this.getParentIndex(currentIndex);
        }
    }

    pop() {
        const result = this.heap[1];
        let currentIndex = 1;
        let leftIndex = 2;
        let rightIndex = 3;

        if(this.heap.length === 2) {
            return this.heap.pop();
        }

        this.heap[1] = this.heap.pop();

        while(this.heap[currentIndex] < this.heap[leftIndex] || this.heap[currentIndex] < this.heap[rightIndex]) {
            if(this.heap[leftIndex] > this.heap[rightIndex]) {
                this.swap(currentIndex, leftIndex);
                currentIndex = leftIndex;
            }
            else {
                this.swap(currentIndex, rightIndex);
                currentIndex = rightIndex;
            }
            leftIndex = this.getLeftChildIndex(currentIndex);
            rightIndex = this.getRightChildIndex(currentIndex);
        }

        return result;
    }
}

const maxHeap = new MaxHeap();

maxHeap.push(5);
maxHeap.push(1);
// maxHeap.push(2);

console.log(maxHeap.heap);
console.log(maxHeap.pop());
console.log(maxHeap.heap);

최소 힙 구현

class MinHeap {
    constructor() {
        this.heap = [null];
    }

    getLeftChildIndex(index) {
        return index * 2;
    }

    getRightChildIndex(index) {
        return (index * 2) + 1;
    }

    getParentIndex(index) {
        return Math.floor(index / 2);
    }
    
    swap(index1, index2) {
        const temp = this.heap[index1];

        this.heap[index1] = this.heap[index2];
        this.heap[index2] = temp;
    }

    isEmpty() {
        return !this.heap[1] ? true : false;
    }

    push(value) {
        if(!value) {
            return;
        }
        this.heap.push(value);

        let currentIndex = this.heap.length - 1;
        let parentIndex = this.getParentIndex(currentIndex);
        
        while(parentIndex !== 0 && this.heap[parentIndex] > value) {
            this.swap(currentIndex, parentIndex);

            currentIndex = parentIndex;
            parentIndex = this.getParentIndex(currentIndex);
        }
    }

    pop() {
        const result = this.heap[1];
        let currentIndex = 1;
        let leftIndex = 2;
        let rightIndex = 3;

        if(this.heap.length === 2) {
            return this.heap.pop();
        }

        this.heap[1] = this.heap.pop();

        while(this.heap[currentIndex] > this.heap[leftIndex] || this.heap[currentIndex] > this.heap[rightIndex]) {
            if(this.heap[leftIndex] < this.heap[rightIndex]) {
                this.swap(currentIndex, leftIndex);
                currentIndex = leftIndex;
            }
            else {
                this.swap(currentIndex, rightIndex);
                currentIndex = rightIndex;
            }
            leftIndex = this.getLeftChildIndex(currentIndex);
            rightIndex = this.getRightChildIndex(currentIndex);
        }

        return result;
    }
}

const minHeap = new MinHeap();

minHeap.push(5);
minHeap.push(1);
// minHeap.push(2);

console.log(minHeap.heap);
console.log(minHeap.pop());
console.log(minHeap.heap);