[BAEKJOON] - 2023번 : 신기한 소수

2023번 문제 링크

해당 문제를 처음 읽었을 때 소수를 판별하기 위한 로직을 짜야 하는 건가라고 생각했지만, 시간 복잡도 차원에서 보면 O(N)의 복잡도의 알고리즘을 사용해야 한다.

일부 필요 없는 조건을 가지치기 한 뒤, DFS로 구현하여 문제를 해결하면 된다.

⌛ 시간 복잡도

• 2초 : 2억
• 제한 조건 : 최대 99,999,999(9천 9백만)
• O(n) 이하 사용 가능

📜 로직

1. 일의 자리인 소수 2,3,5,7을 분기로 dfs 수행
2. 십의 자리 수부터는 뒤의 숫자를 붙일 때, 짝수는 2의 약수가 무조건 들어가기 때문에 '가지치기' 해준다.(따라서 홀수만 뒤에 숫자로 붙여준다)

ex)

• 2 + '홀수' : 소수일수도 아닐수도 있다.
• 2 + '짝수' : 약수에 2가 무조건 포함되어 소수가 아니다.

3. 값을 붙여준 뒤, 해당 값이 소수인지 판별해준다.
4. 소수가 맞으면, 값을 붙여준 뒤 자릿수를 1자리 올려준다.
   • 소수 판별 시, 에라토스테네스 체 의 원리를 이용한다.
   • 2 ~ √n 까지의 수만 판별해준다.('가지치기')
5. 자릿수가 일치하면 소수여부 판별 후 출력한다.

위의 로직에서 에라토스테네스 체를 사용하지 않았을 때와 사용했을 때의 시간차이는 무려 600ms나 차이난다.

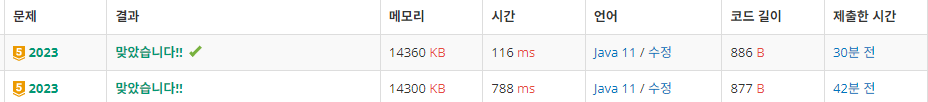
😀 성공

적용 전

import java.io.*;
import java.util.*;

public class Main{
    static int N;
    public static void main(String[] args)throws IOException{
        BufferedReader br = new BufferedReader(new InputStreamReader(System.in));
        N = Integer.parseInt(br.readLine());
        br.close();
        
        dfs(2,1);
        dfs(3,1);
        dfs(5,1);
        dfs(7,1);
    }
    
    static void dfs(int n, int digit){
        if(N == digit){
            if(isPrime(n)){
                System.out.println(n);
            }
            return;
        }
        
        for(int i=1; i<10; i+=2){
            if(isPrime(n*10+i)){
                dfs(n*10+i,digit+1);
            }
        }
    }
    
    static boolean isPrime(int n){
        for(int i=2; i <= n/2; i++){
            if(n%i==0){
                return false;
            }
        }
        return true;
    }
}

적용 후

import java.io.*;
import java.util.*;

public class Main{
    static int N;
    public static void main(String[] args)throws IOException{
        BufferedReader br = new BufferedReader(new InputStreamReader(System.in));
        N = Integer.parseInt(br.readLine());
        br.close();
        
        dfs(2,1);
        dfs(3,1);
        dfs(5,1);
        dfs(7,1);
    }
    
    static void dfs(int n, int digit){
        if(N == digit){
            if(isPrime(n)){
                System.out.println(n);
            }
            return;
        }
        
        for(int i=1; i<10; i+=2){
            if(isPrime(n*10+i)){
                dfs(n*10+i,digit+1);
            }
        }
    }
    
    static boolean isPrime(int n){
        for(int i=2; i <= Math.sqrt(n); i++){
            if(n%i==0){
                return false;
            }
        }
        return true;
    }
}