분할 정복 개념 및 연습문제

분할 정복(Divide and Conquer)

큰 문제를 작은 부분 문제로 나누어 해결하는 방법

• 합병 정렬, 퀵 정렬, 이진 검색, ...

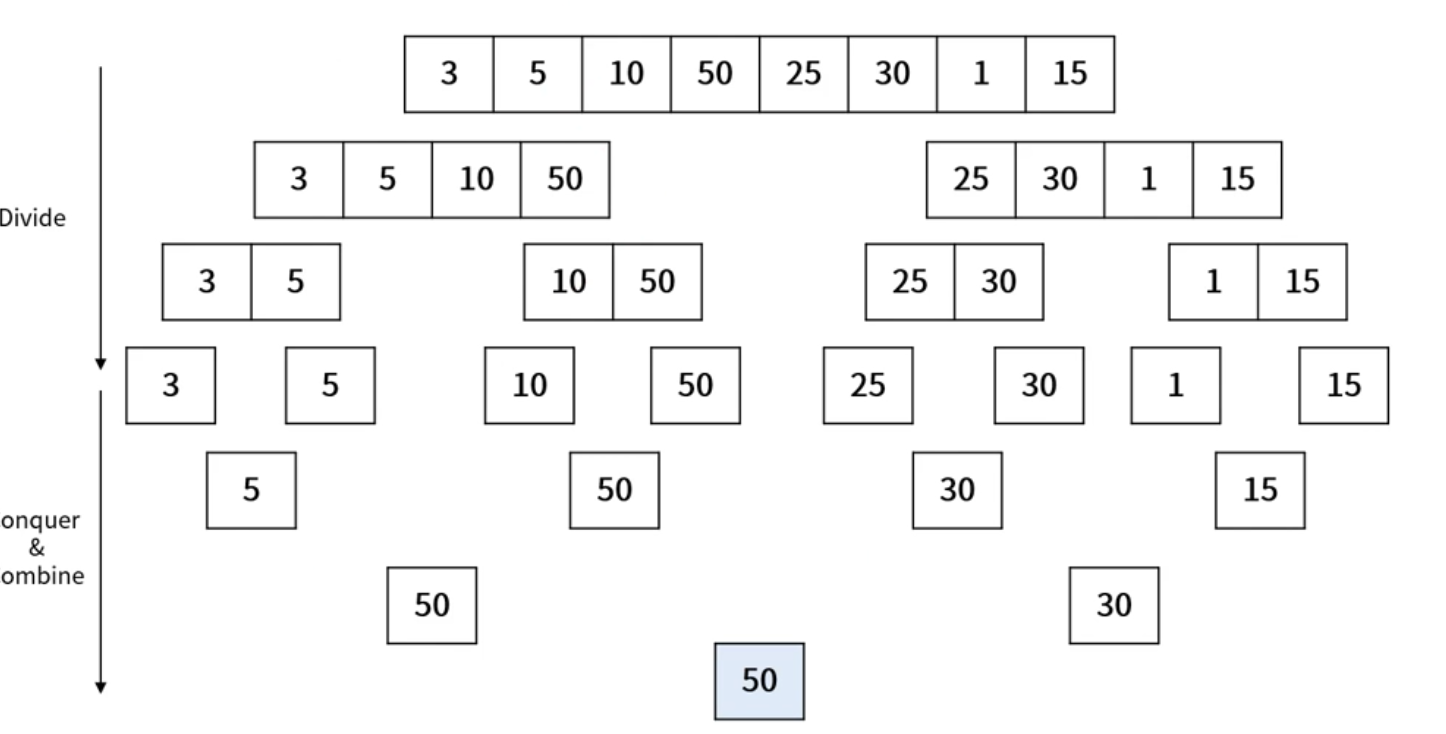
분할 정복 과정
1. 문제를 하나 이상의 작은 부분들로 분할
2. 부분들을 각각 정복
3. 부분들의 해답을 통합하여 원래 문제의 답을 구함

장점/단점

문제를 나누어 처리하며 어려운 문제 해결 가능

병렬 처리에 이점이 있음

메모리를 많이 사용(재귀 호출 구조)

예시

최대 값 찾기

코드 예시

public static int getMax(int[] arr, int left, int right){
	int m = (left+right) / 2;
    if(left == right) return arr[left];
    
    left = getMax(arr,left,m);
    right = getMax(arr, m+1, right);
    
    return(left> right)? left:right; // conquer
}

public class Main {

    public static int getMax(int[] arr, int left, int right) {

        int m = (left+right) / 2;
        if(left == right){
            return arr[left];
        }

        left = getMax(arr, left, m);
        right = getMax(arr,m+1,right);

        return (left>right)? left:right;
    }


    public static void main(String[] args) {
        int arr[] = {6, 2, 9, 8, 1, 4, 17, 5};
        System.out.println(getMax(arr, 0, arr.length - 1));
    }
}

연습문제

문제1

// Practice1
// 정수형 배열 nums 가 주어졌다.
// 연속된 부분 배열의 합 중 가장 큰 값을 출력하세요.

// 입출력 예시
// nums: -5, 0, -3, 4, -1, 3, 1, -5, 8
// 출력: 10

// nums: 5, 4, 0, 7, 8
// 출력: 24

// 투 포인터 양끝에 놓는 것도 좋음(이동 조건 잘 세워야, 이 경우엔 분기 조건이 좀 많음)
public class Practice1 {

    public static int solution(int[] nums) {
        if (nums == null || nums.length == 0) {
            return 0;
        }

        return divideSubArray(nums,0,nums.length-1);
    }

    public static int divideSubArray(int[] nums, int left, int right){
        if(left == right){
            return nums[left];
        }

        int mid = left + (right-left)/2;
        int maxLeft = divideSubArray(nums,left,mid);
        int maxRight = divideSubArray(nums,mid+1,right);

        int maxArr = getMaxSubArray(nums,left,mid,right);
        return Math.max(maxLeft, Math.max(maxRight, maxArr));
    }

    public static int getMaxSubArray(int[] nums, int left, int mid, int right){
        int sumLeft = 0;
        int maxLeft = Integer.MIN_VALUE;

        for (int i = mid; i >= left; i--) {
            sumLeft += nums[i];
            maxLeft = Math.max(maxLeft, sumLeft);
        }

        int sumRight = 0;
        int maxRight = Integer.MIN_VALUE;
        for (int i = mid+1; i <= right; i++) {
            sumRight += nums[i];
            maxRight = Math.max(maxRight, sumRight);
        }

        return maxLeft + maxRight;
    }


    public static void main(String[] args) {
        // Test code
        int[] nums = {-5, 0, -3, 4, -1, 3, 1, -5, 8};
        System.out.println(solution(nums));

        nums = new int[]{5, 4, 0, 7, 8};
        System.out.println(solution(nums));
    }
}

문제 2

// Practice2
// 2차원 정수형 배열 lists 가 주어졌다.
// lists[i] 에는 각 링크드 리스트의 원소 정보가 들어 있고,
// 원소들은 오름차순 정렬된 상태이다.
// 모든 링크드 리스트를 하나의 정렬된 링크드 리스트로 합병하세요.

// 입출력 예시
// lists: {{2, 3, 9}, {1, 5, 7}, {3, 6, 7, 11}}
// 출력: 1 -> 2 -> 3 -> 3 -> 5 -> 6 -> 7 -> 7 -> 9 -> 11


class Node {
    int val;
    Node next;

    Node(int val) {
        this.val = val;
        this.next = null;
    }
}

public class Practice2 {
    public static Node solution(Node[] lists) {
        if (lists == null || lists.length == 0) {
            return null;
        }

        return divideList(lists, 0, lists.length-1);
    }

    public static Node divideList(Node[] lists, int left, int right){
        if(left == right){
            return lists[left];
        }

        int mid = left + (right-left) / 2;
        Node l1 = divideList(lists,left,mid);
        Node l2 = divideList(lists,mid+1,right);

        return mergeList(l1, l2);
    }

    public static Node mergeList(Node l1, Node l2){
        if(l1 == null){
            return l2;
        }

        if(l2 == null){
            return l1;
        }

        Node merge = new Node(0);
        Node cur = merge;

        while(l1 != null && l2 != null){
            if(l1.val < l2.val){
                cur.next = l1;
                l1 = l1.next;
            }else{
                cur.next = l2;
                l2 = l2.next;
            }
            cur = cur.next;
        }
        if(l1 != null){
            cur.next = l1;
        }

        if(l2 != null){
            cur.next = l2;
        }

        return merge.next;
    }



    // 문제에 주어진 2차원 배열을 링크드 리스트로 구성
    public static void setUpLinkedList(Node[] node, int[][] lists) {
        for (int i = 0; i < lists.length; i++) {
            node[i] = new Node(lists[i][0]);
        }

        for (int i = 0; i < lists.length; i++) {
            Node cur = node[i];
            for (int j = 1; j < lists[i].length; j++) {
                cur.next = new Node(lists[i][j]);
                cur = cur.next;
            }
        }
    }

    // 결과 출력 부분
    public static void printList(Node node) {
        Node cur = node;
        while (cur.next != null) {
            System.out.print(cur.val + " -> ");
            cur = cur.next;
        }
        System.out.println(cur.val);
    }

    public static void main(String[] args) {
        // Test code
        int[][] lists = {{2, 3, 9}, {1, 5, 7}, {3, 6, 7, 11}};
        Node[] node = new Node[lists.length];
        setUpLinkedList(node, lists);
        Node combinedNode = solution(node);
        printList(combinedNode);
    }
}