Algorithm 21일차

알고리즘에서 이항 계수를 구하는 데 DP를 사용할 수 있다.

nCk는 n-1Ck-1 + n-1Ck로 정의된다. 따라서 nCk를 구하는 재귀 함수는 아래와 같다.

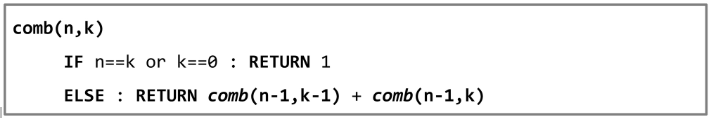
위 함수는 순수 함수이다.

이 때, 아래처럼 호출의 중복이 일어난다.

따라서 이를 해결하고자 메모이제이션을 활용할 수 있다. 이를 활용한 코드는 아래와 같다.

import java.io.BufferedReader;
import java.io.InputStreamReader;
import java.util.StringTokenizer;

public class KnapsackTest {
	
	public static void main(String[] args) throws Exception {
		BufferedReader br = new BufferedReader(new InputStreamReader(System.in));
		
		int[][] arr = new int[31][31];
		
		for (int i = 1; i <= 30; i++) {
			arr[i][i] = 1;
			arr[i][1] = i;
		}
		
		for (int i = 3; i <= 30; i++) {
			for (int j = 2; j < i; j++) {
				arr[i][j] = arr[i-1][j-1] + arr[i-1][j];
			}
		}
		
		
		StringTokenizer st = new StringTokenizer(br.readLine());
			
		int M = Integer.parseInt(st.nextToken());
		int N = Integer.parseInt(st.nextToken());
			
		System.out.println(arr[M][N]);
	}
}

입력값은 아래와 같다.

30 15

출력 결과는 아래와 같다.

155117520

이러면 일반 조합으로는 무리가 있던 30C15를 무리 없이 구할 수 있다.

알고리즘에서 동전 거스름돈을 구할 때 DP를 사용할 수 있다.

동전 거스름돈을 주는 문제 정의는 아래와 같다.

최소한의 동전을 사용해 고객에게 거스름돈을 준다.

이는 동전들이 배수가 아니라면 탐욕 기법으로 문제를 풀 수 없다.
따라서 DP로 이를 풀어보면 아래와 같다.

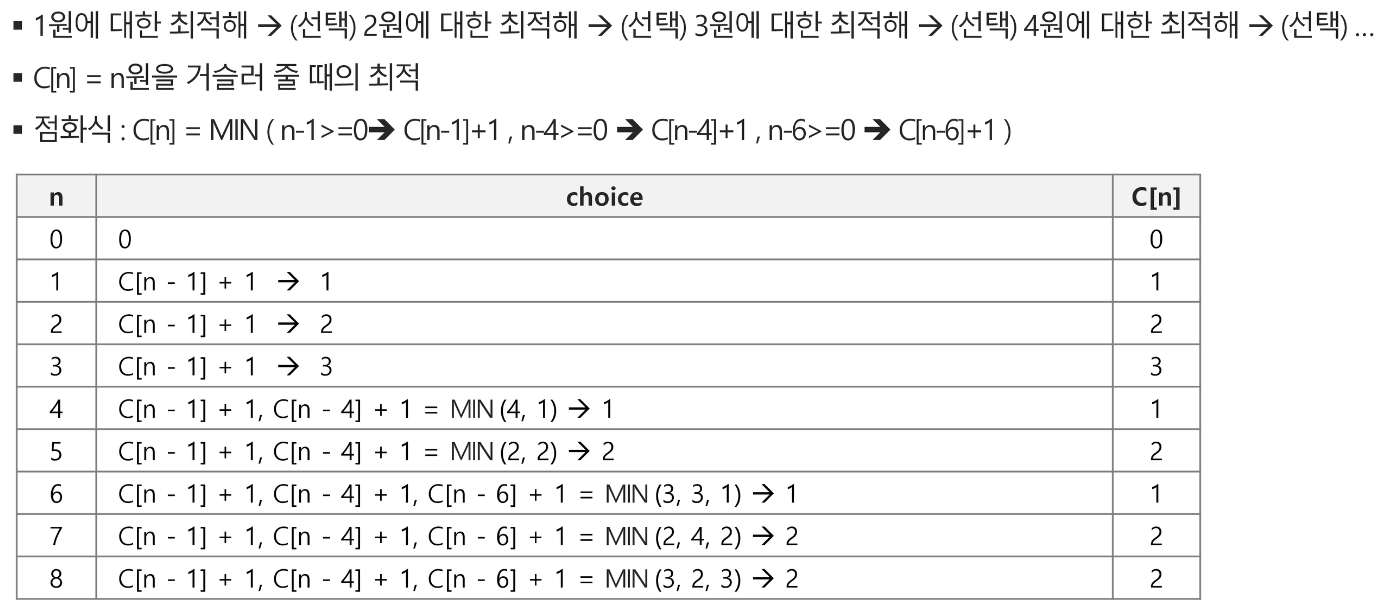
동전 거스름돈 문제를 실제로 구현하면 아래와 같다.

import java.io.BufferedReader;
import java.io.InputStreamReader;
import java.util.Arrays;
import java.util.StringTokenizer;

public class Coin {
	
	public static void main(String[] args) throws Exception {
		BufferedReader br = new BufferedReader(new InputStreamReader(System.in));
		int target = Integer.parseInt(br.readLine());
		
		int N = Integer.parseInt(br.readLine());
		
		int[] arr = new int[N];
		StringTokenizer st = new StringTokenizer(br.readLine());
		
		for (int i = 0; i < N; i++) {
			arr[i] = Integer.parseInt(st.nextToken());
		}
		
		Arrays.sort(arr);
		
		int[] dynamic = new int[target+1];
		Arrays.fill(dynamic, Integer.MAX_VALUE);
		dynamic[0] = 0;
		
		for (int i = 1; i <= target; i++) {
			int threshold = 0;
			
			for (int j = 0; j < N; j++) {
				if (i >= arr[j]) {
					threshold = j;
					continue;
				}
				
				break;
			}
			
			for (int j = 0; j <= threshold; j++) {
				dynamic[i] = Math.min(dynamic[i], dynamic[i - arr[j]] + 1);
			}
		}
		
		System.out.println(dynamic[target]);
	}
}

입력값은 아래와 같다.

8
3
1 4 6

출력 결과는 아래와 같다.

2