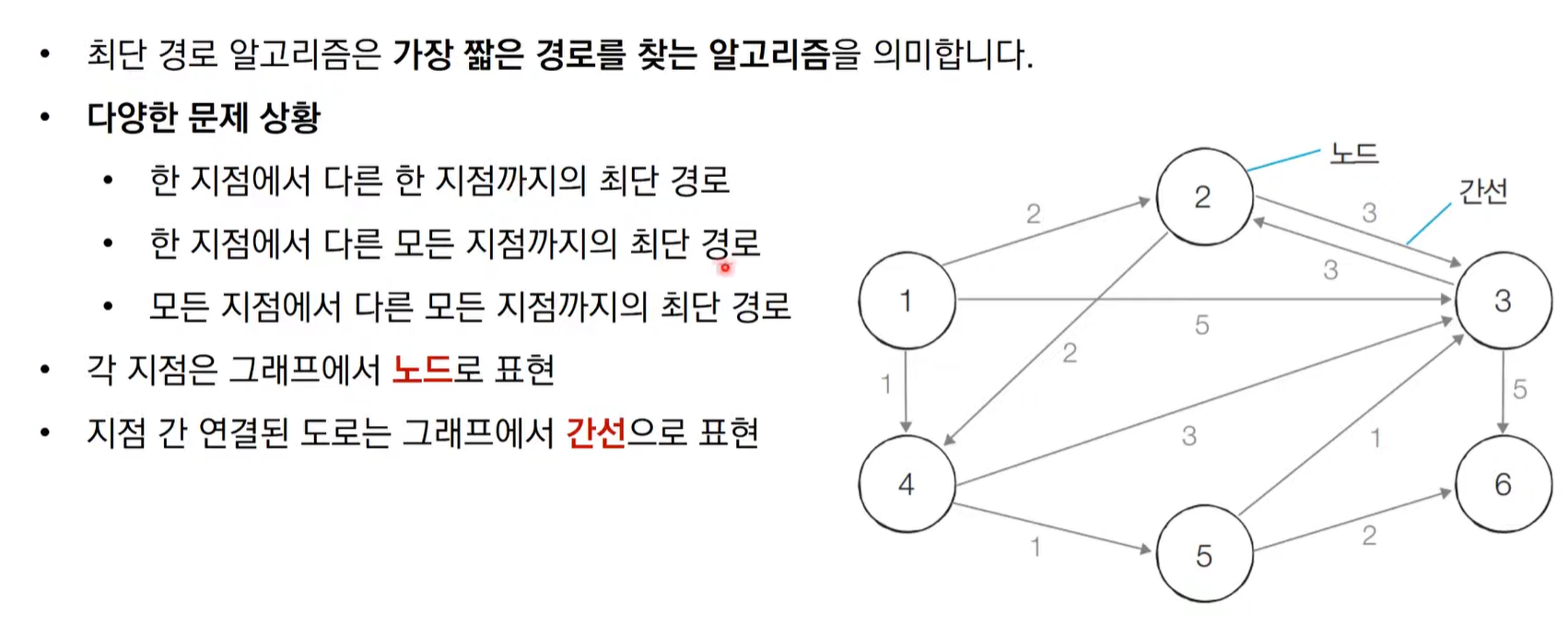
최단 경로 문제

• 가장 짧은 경로를 찾는 알고리즘
• 각 지점은 그래프에서 노드로 표현
• 지점 간 연결된 도로는 그래프에서 간선으로 표현

다익스트라 알고리즘

• 특정한 노드에서 출발하여 다른 모든 노드로 가는 최단 경로 계산
• 음의 간선이 없을 때 정상적으로 작당
• 다익스트라 알고리즘은 그리디 알고리즘으로 분류
  • 매 상황에서 가장 비용이 적은 노드를 선택해 임의의 과정을 반복

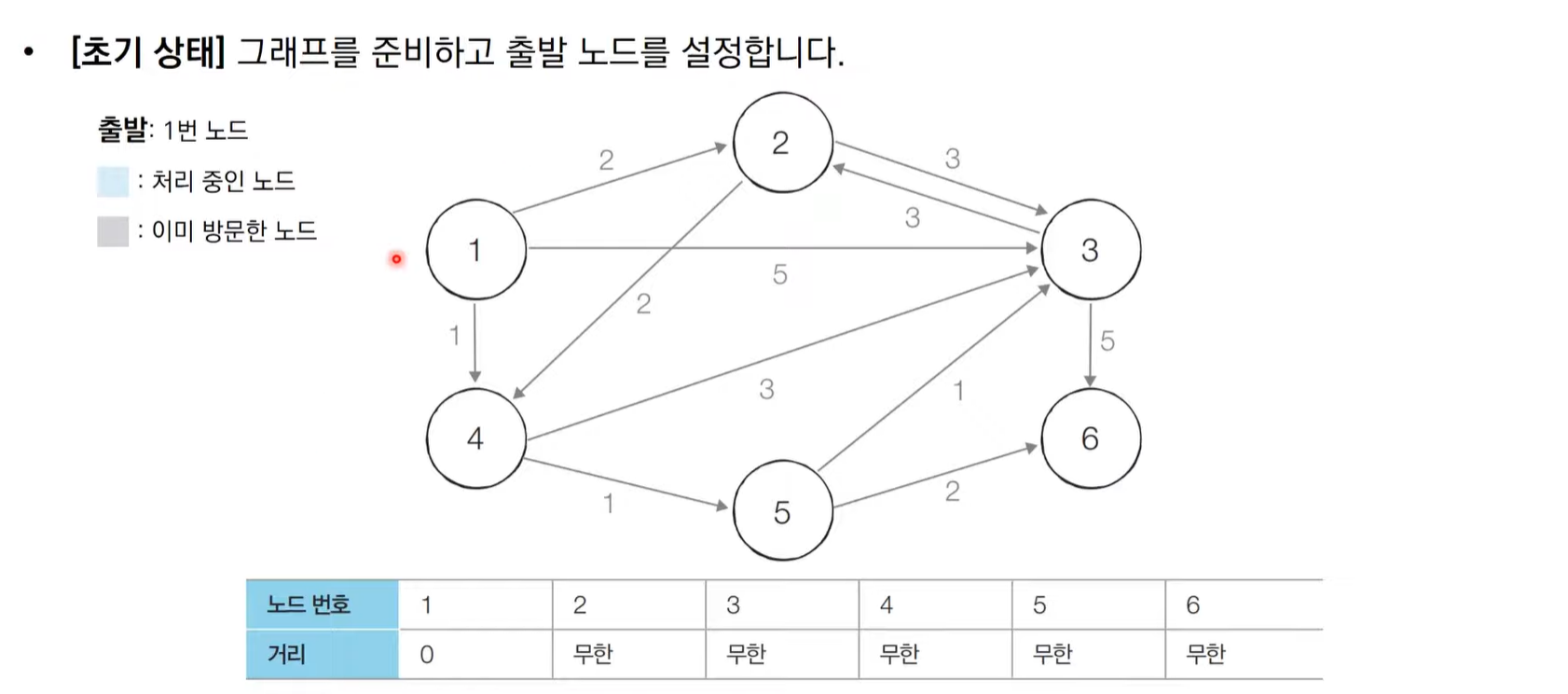
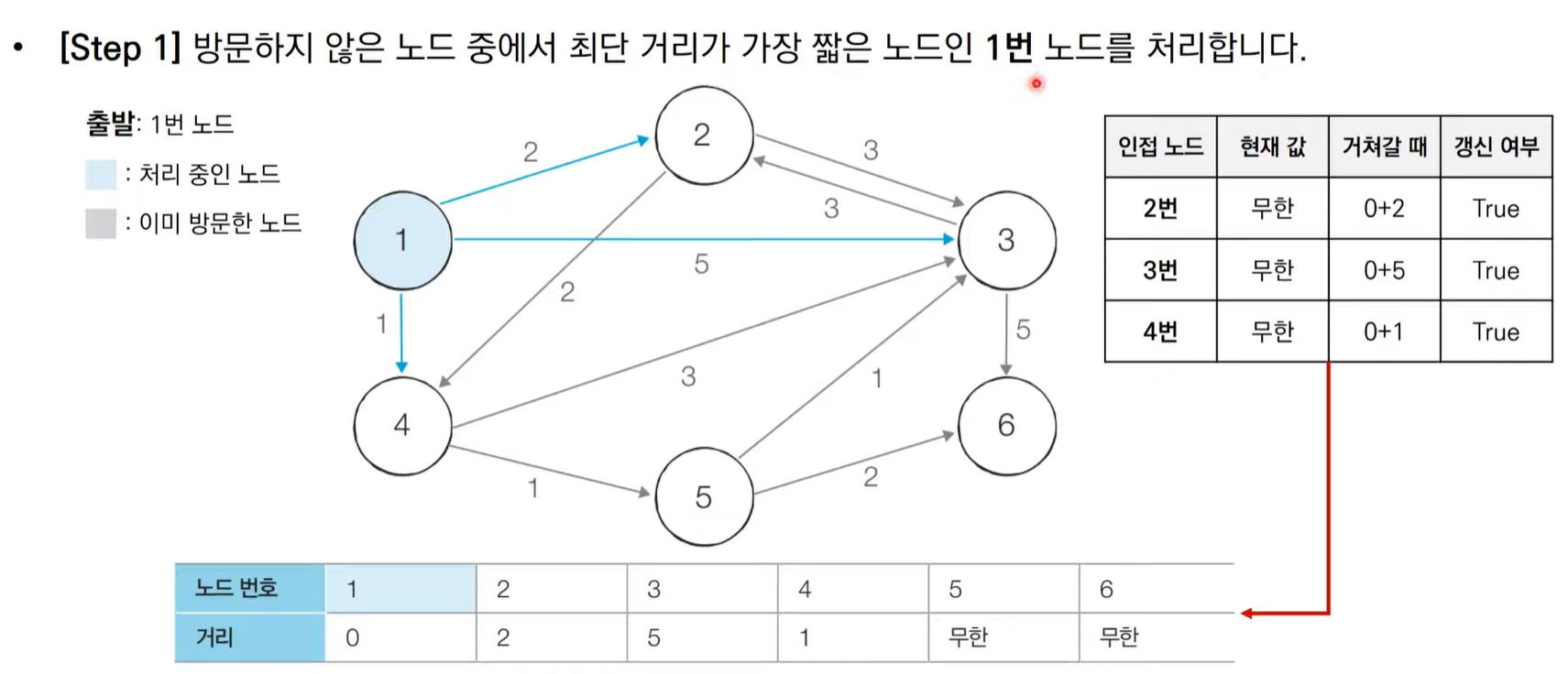
다익스트라 알고리즘 동작 과정

다익스트라 알고리즘 동작 과정 살펴보기

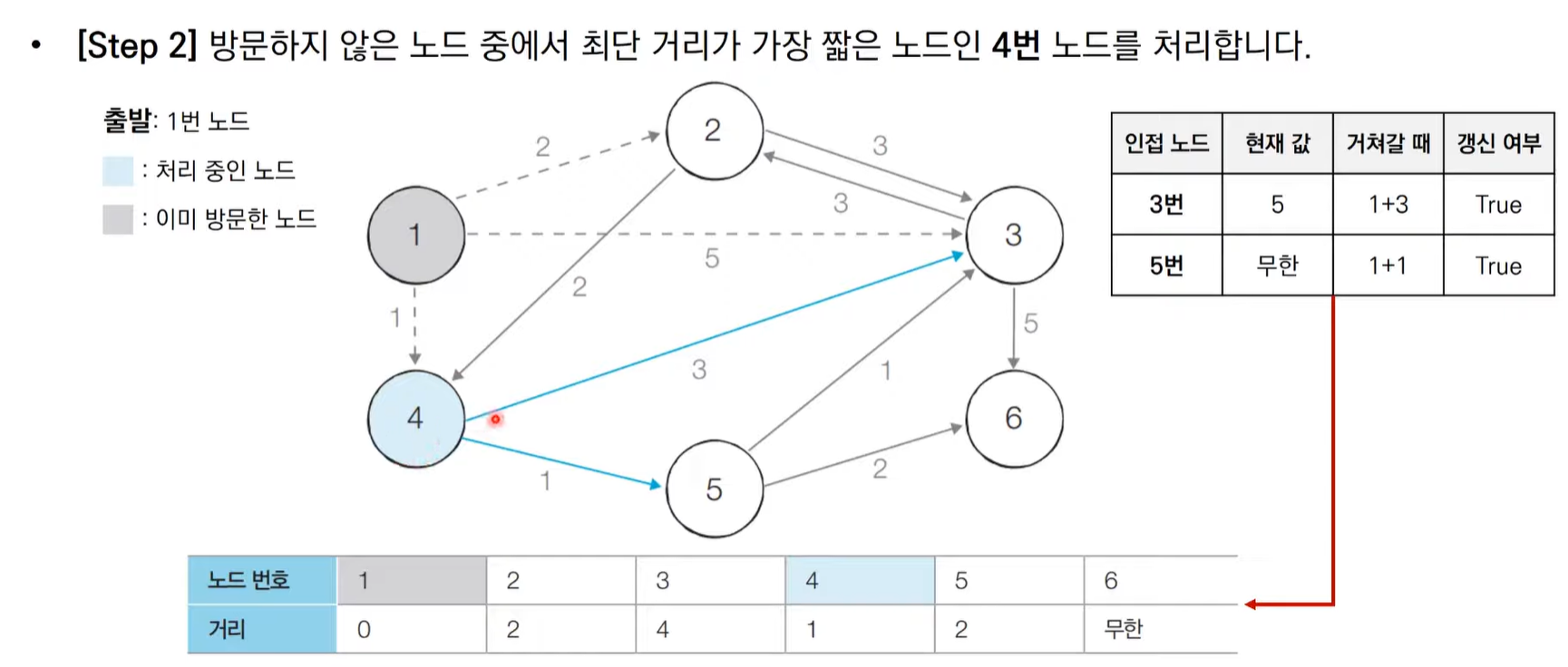
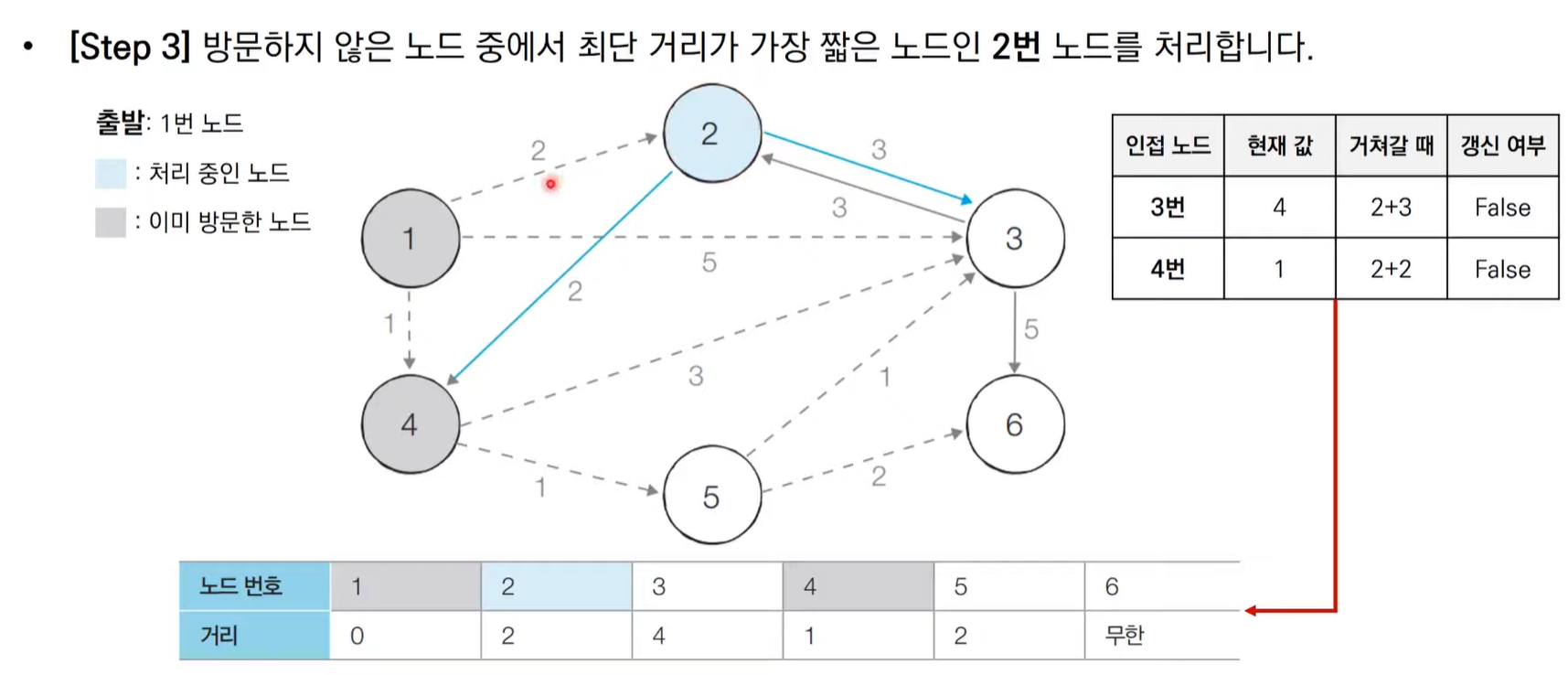
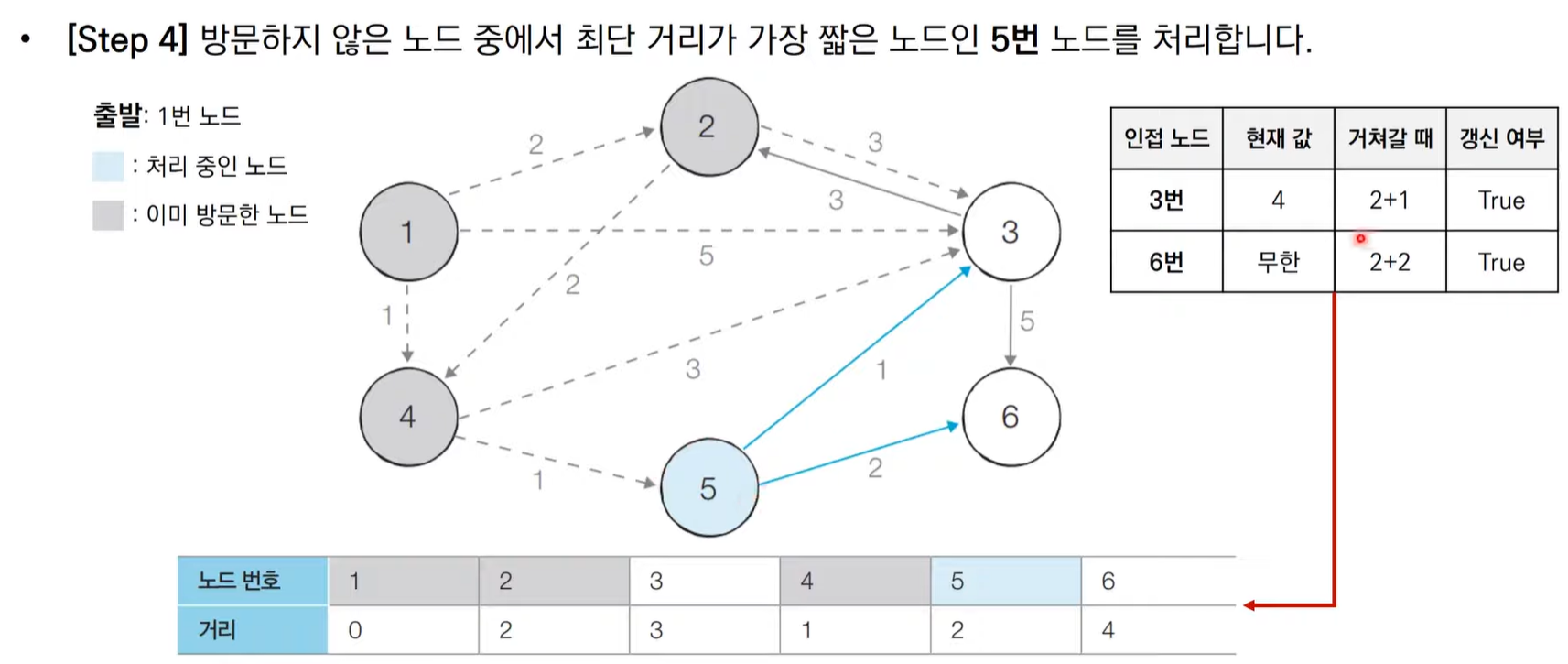
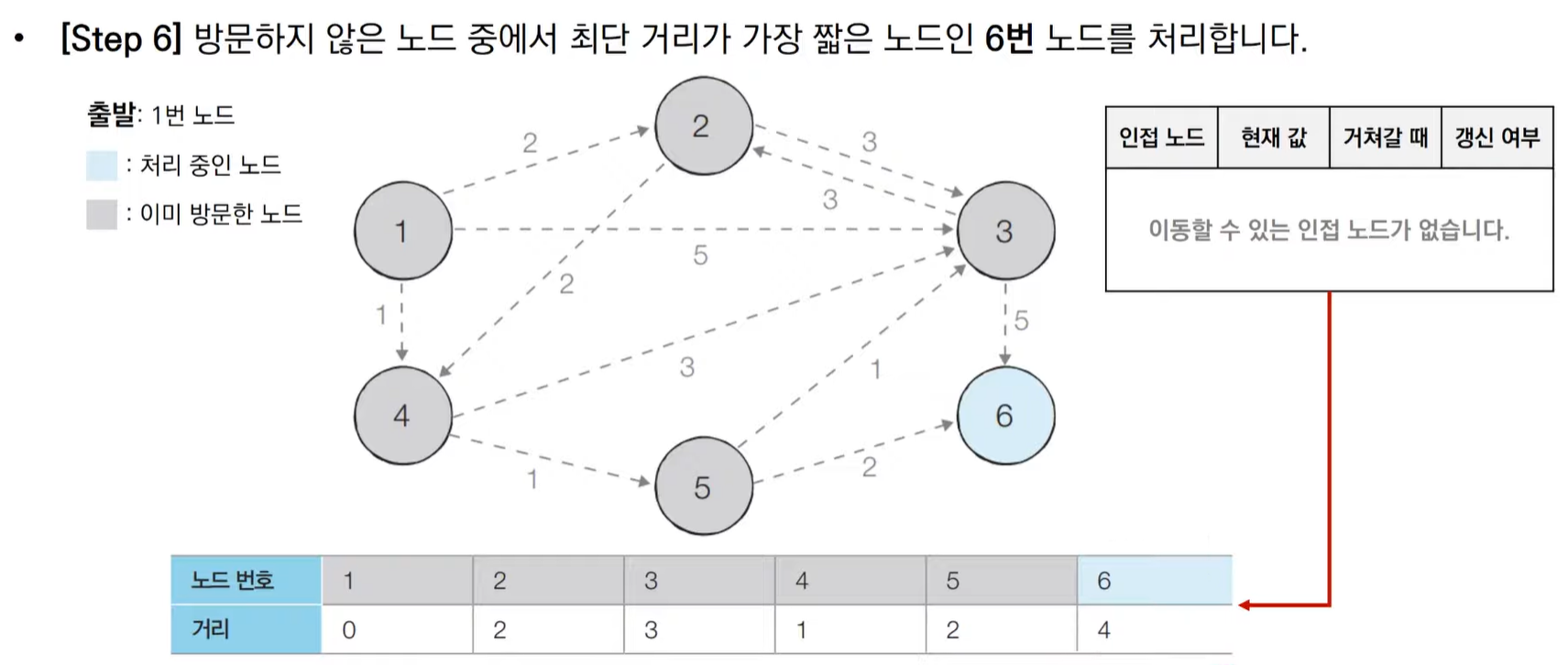
다익스트라 알고리즘 특징

• 그리디 알고리즘: 매 상황에서 방문하지 않은 가장 비용이 적은 노드를 선택

• 단계를 거치며 한 번 처리된 노드의 최단 거리는 고정되어 더 이상 바뀌지 않음
  • 한 단계당 하나의 노드에 대한 최단 거리를 확실히 찾는 것으로 이해할 수 있음
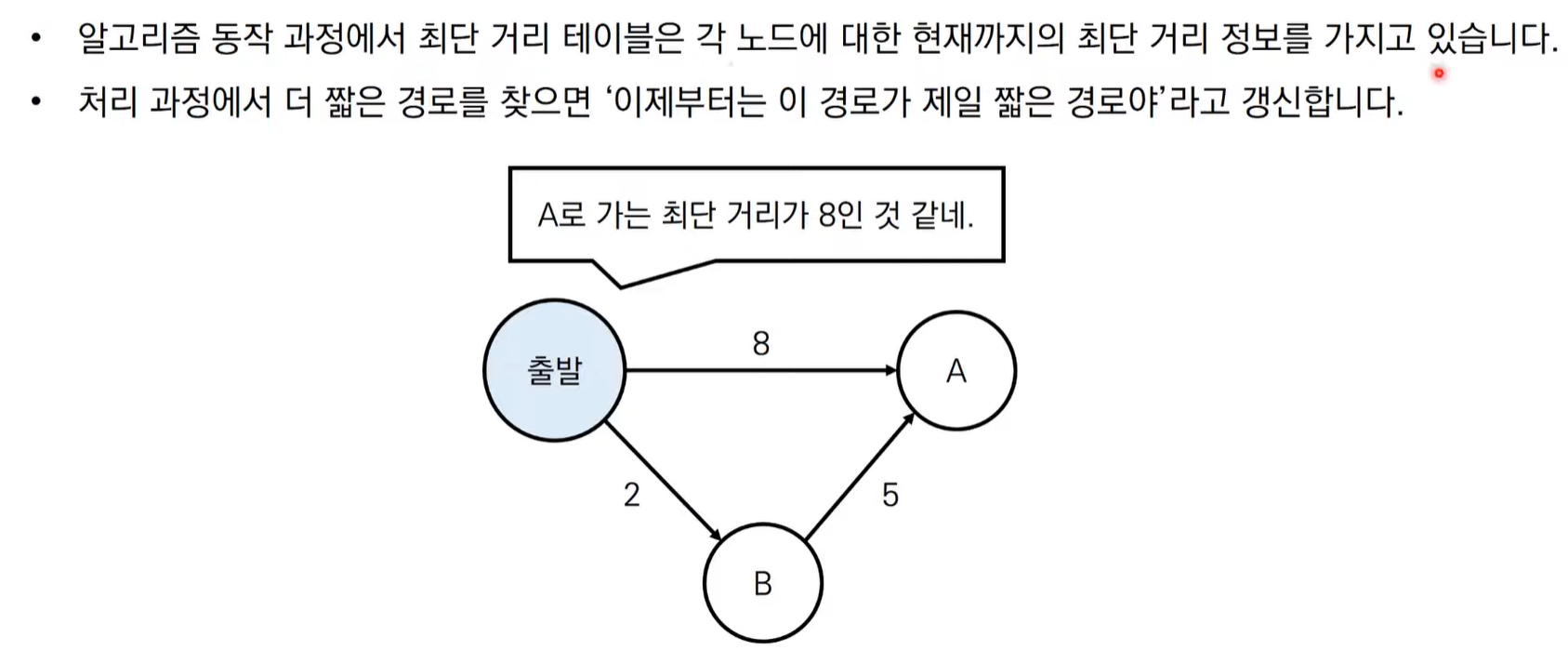
• 다익스트라 알고리즘을 수행한 뒤 테이블에 각 노드까지의 최단 거리 정보가 저장
  • 완벽한 형태의 최단 경로를 구하려면 소스코드에 추가적인 기능을 더 넣어야 함

다익스트라 알고리즘 간단한 구현 방법

• 단계마다 방문하지 않은 노드 중에서 최단 거리가 가장 짧은 노드를 선택하기 위해 매 단계마다 1차원 테이블의 모든 원소를 확인(순차 탐색)합니다.

import sys
input = sys.stdin.readline
INF = int(1e9)

n,m = map(int,input().split())
start = int(input())
graph = [[] for i in range(n+1)]
visit = [False] * (n+1)
distance = [INF] * (n+1)

for _ in range(m):
    a,b,c = map(int,input().split())
    # a번 노드에서 b번 노드로 가는 비용이 c라는 의미
    graph[a].append((b,c))

# 방문하지 않은 노드 중에서 가장 최단 거리가 짧은 노드의 번호를 반환
def get_smallest_node():
    min_value = INF
    idx = 0 # 가장 최단 거리가 짧은 노드(인덱스)
    for i in range(1,n+1):
        if distance[i] < min_value and not visit[i]:
            min_value = distance[i]
            idx = i
    return idx

def dijkstra(start):
    # 시작 노드에 대해서 초기화
    distance[start] = 0
    visit[start] = True
    
    for j in graph[start]:
        distance[j[0]] = j[1]
    
    # 시작 노드를 제외한 전체 n-1개의 노드에 대해 반복
    for i in range(n-1):
        # 현재 최단 거리가 가장 짧은 노드를 꺼내서 방문처리
        now = get_smallest_node
        visit[now] = True
    
        # 현재 노드와 연결된 다른 노드를 확인
        for j in graph[now]:
            cost = distance[now] + j[i]
            # 현재 노드를 거쳐서 다른 노드로 이동하는 거리가 더 짧은 경우
            if cost < distance[j[0]]:
                distance[j[0]] = cost

dijkstra(start)

for i in range(1,n+1):
    if distance[i] == INF:
        print("INFINITY")
    else:
        print(distance[i])

다익스트라 알고리즘 성능 분석

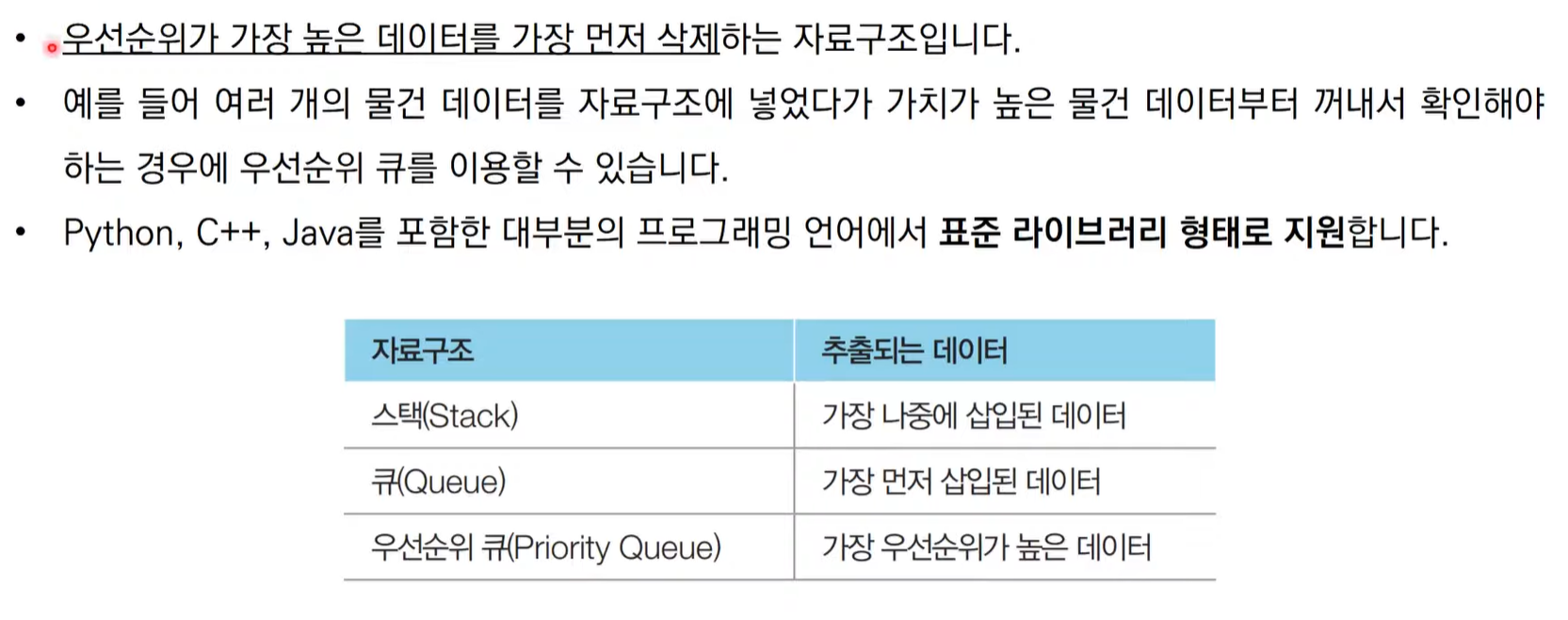
우선순위 큐(Prioriy Queue)

• 우선 순위가 가장 높은 데이터를 가장 먼저 삭제하는 자료구조
• 여러 개의 물건 데이터를 자료구조에 넣었다가 가치가 높은 물건 데이터부터 꺼내서 확인해야 하는 경우에 우선순위 큐를 이용

힙(Heap)

• 우선순위 큐를 구현하기 위해 사용하는 자료구조 중 하나
• 최소 힙(Min Heap)과 최대 힙(Max Heap)
• 다익스트라 최단 경로 알고리즘을 포함해 다양한 알고리즘에서 사용

힙 라이브러리 사용 예제: 최소 힙

import heapq

def heapsort(iterable):
    h = []
    result = []
    # 모든 원소를 차례대로 힙에 삽입
    for value in iterable:
        heapq.heappush(h,value)
    # 힙에 삽입된 모든 원소를 차례대로 꺼내어 담기
    for i in range(len(h)):
        result.append(heapq.heappop(h))
    
    return result

result = heapsort([1,3,5,7,9,2,4,6,8,0])
print(result)

힙 라이브러리 사용 예제: 최대 힙

import heapq

def heapsort(iterable):
    h = []
    result = []
    # 모든 원소를 차례대로 힙에 삽입
    for value in iterable:
        heapq.heappush(h,-value)
    # 힙에 삽입된 모든 원소를 차례대로 꺼내어 담기
    for i in range(len(h)):
        result.append(-heapq.heappop(h))
    
    return result

result = heapsort([1,3,5,7,9,2,4,6,8,0])
print(result)

다익스트라 알고리즘 - 개선된 구현 방법

• 단계마다 방문하지 않은 노드 중에서 최단 거리가 가장 짧은 노드를 선택하기 위해 힙(Heap) 자료구조 이용
• 다익스트라 알고리즘이 동작하는 기본 원리는 동일
  • 현재 가장 가까운 노드를 저장해 놓기 위해서 힙 자료구조를 추가적으로 이용
  • 현재의 최단 거리가 가장 짧은 노드를 선택해야 하므로 최소 힙을 사용

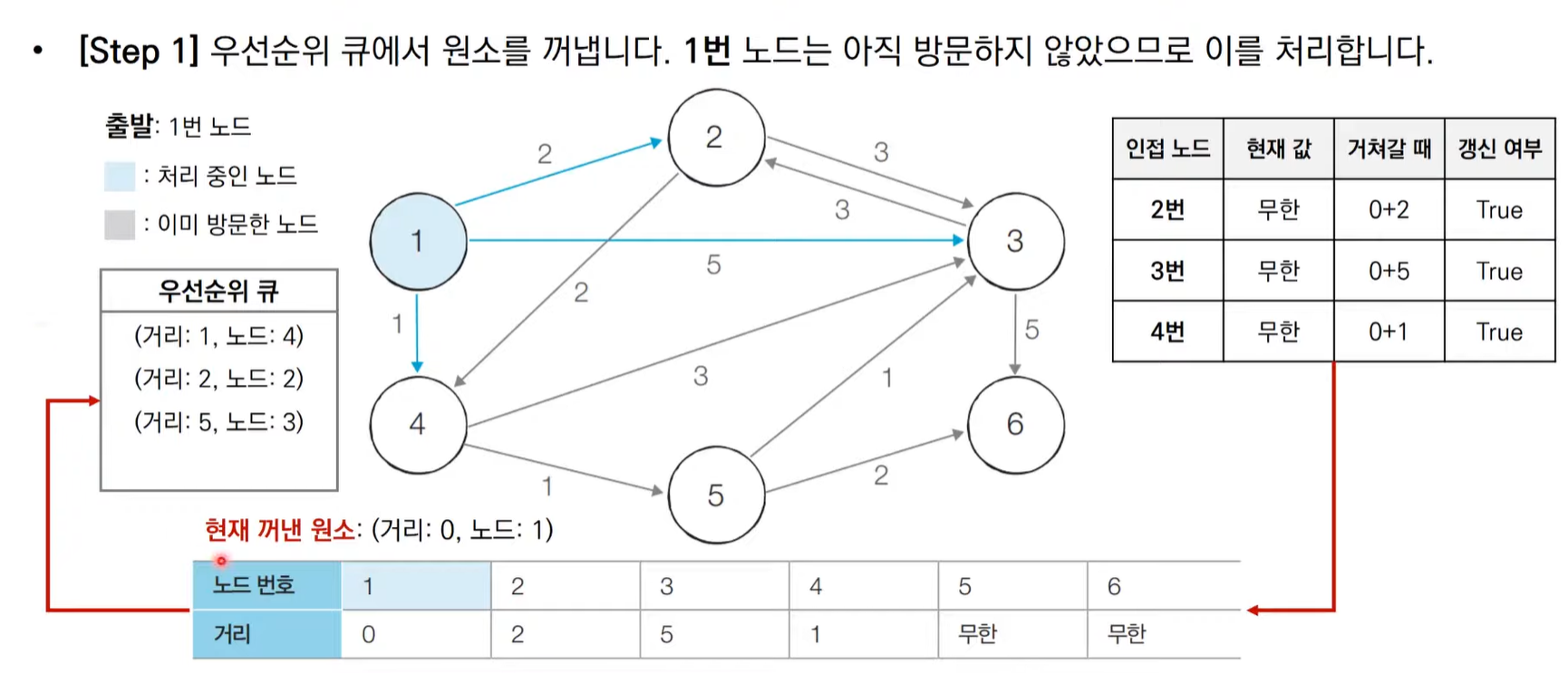
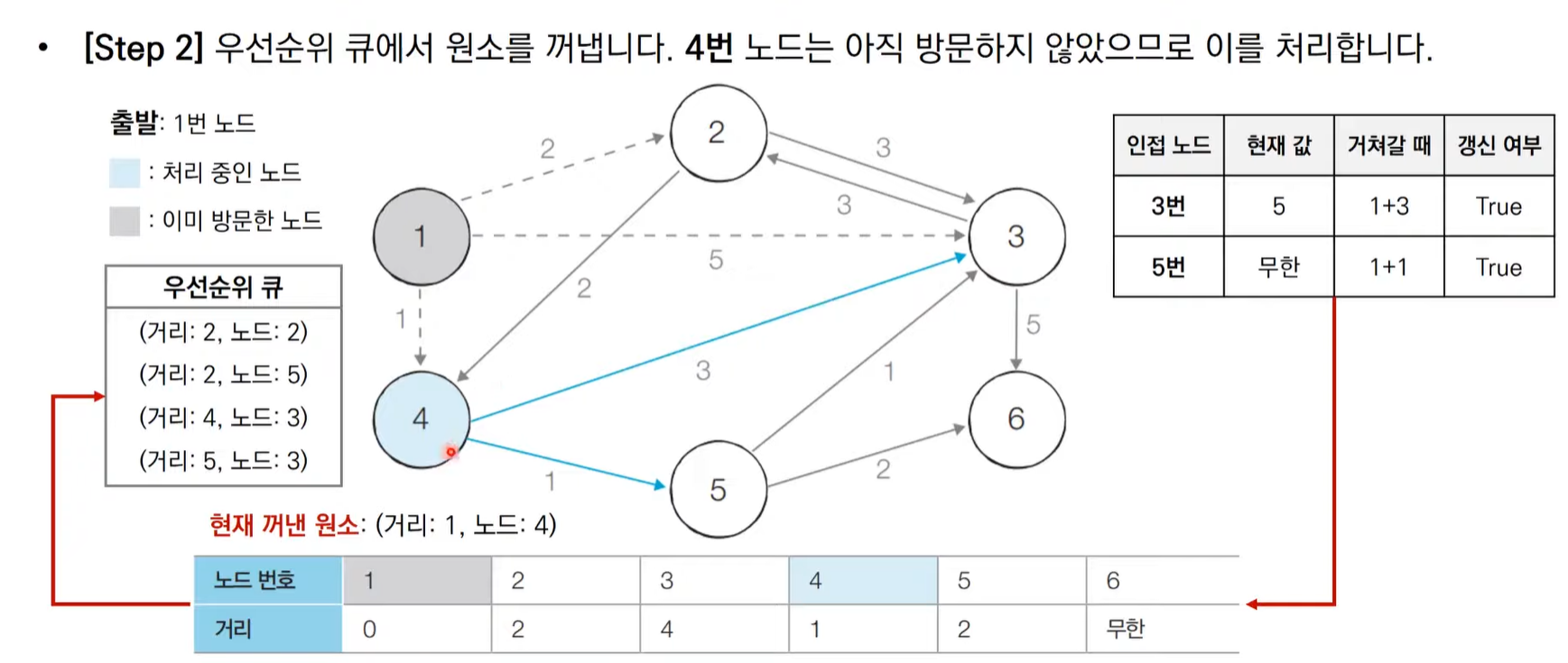
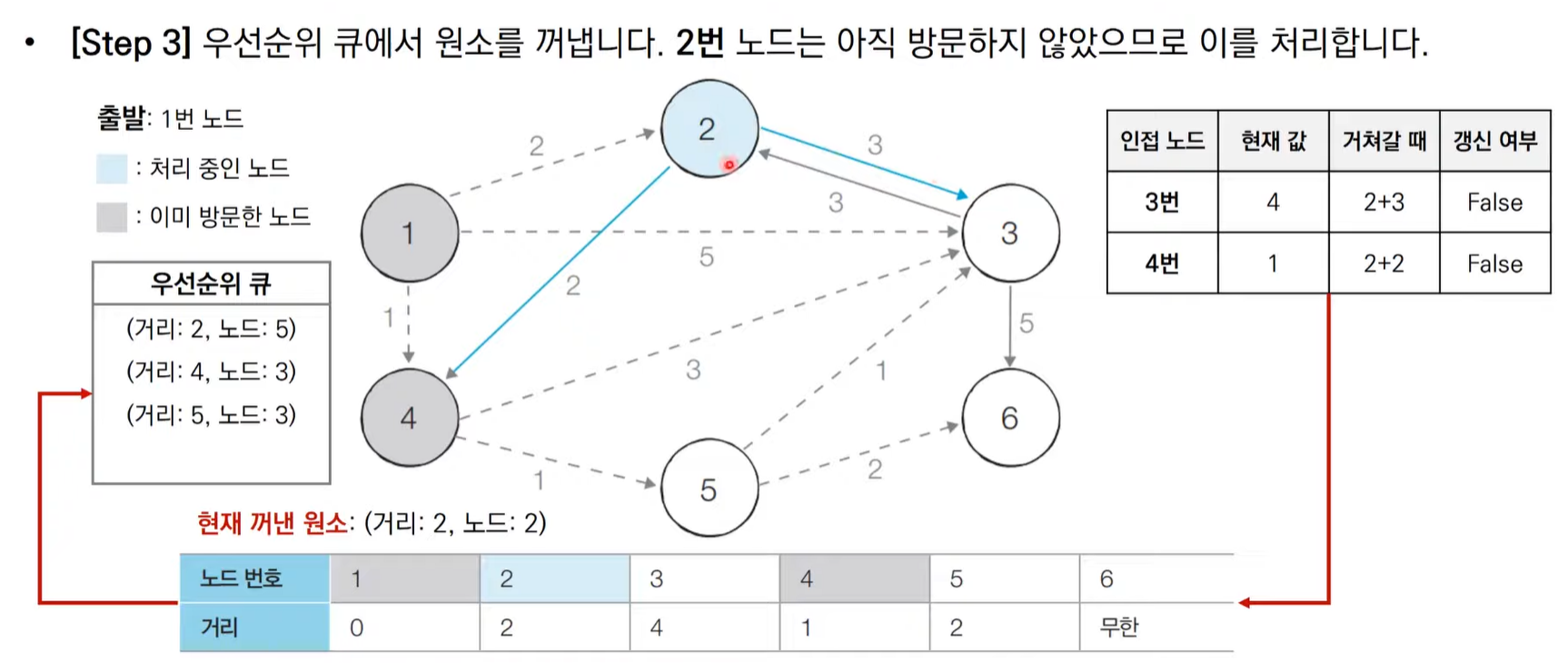
다익스트라 알고리즘 동작 과정 살펴보기(우선순위 큐)

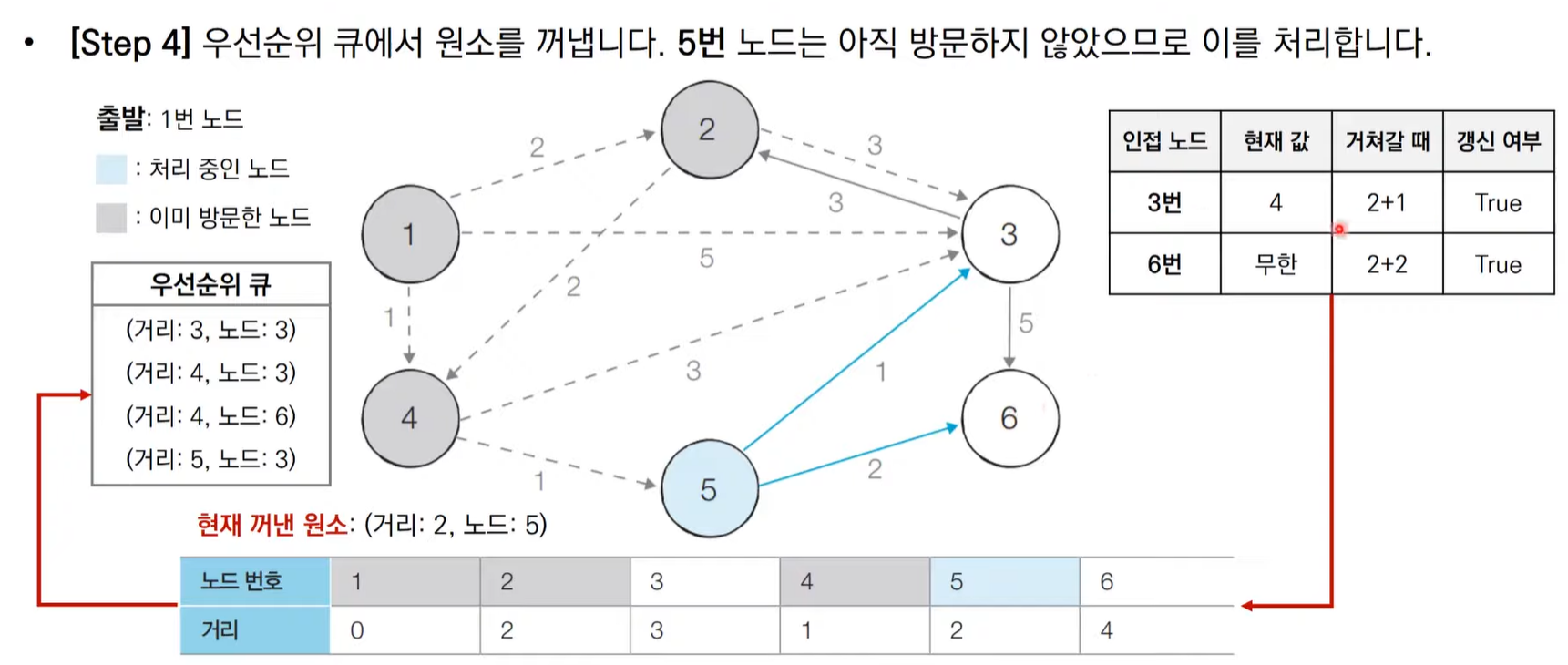
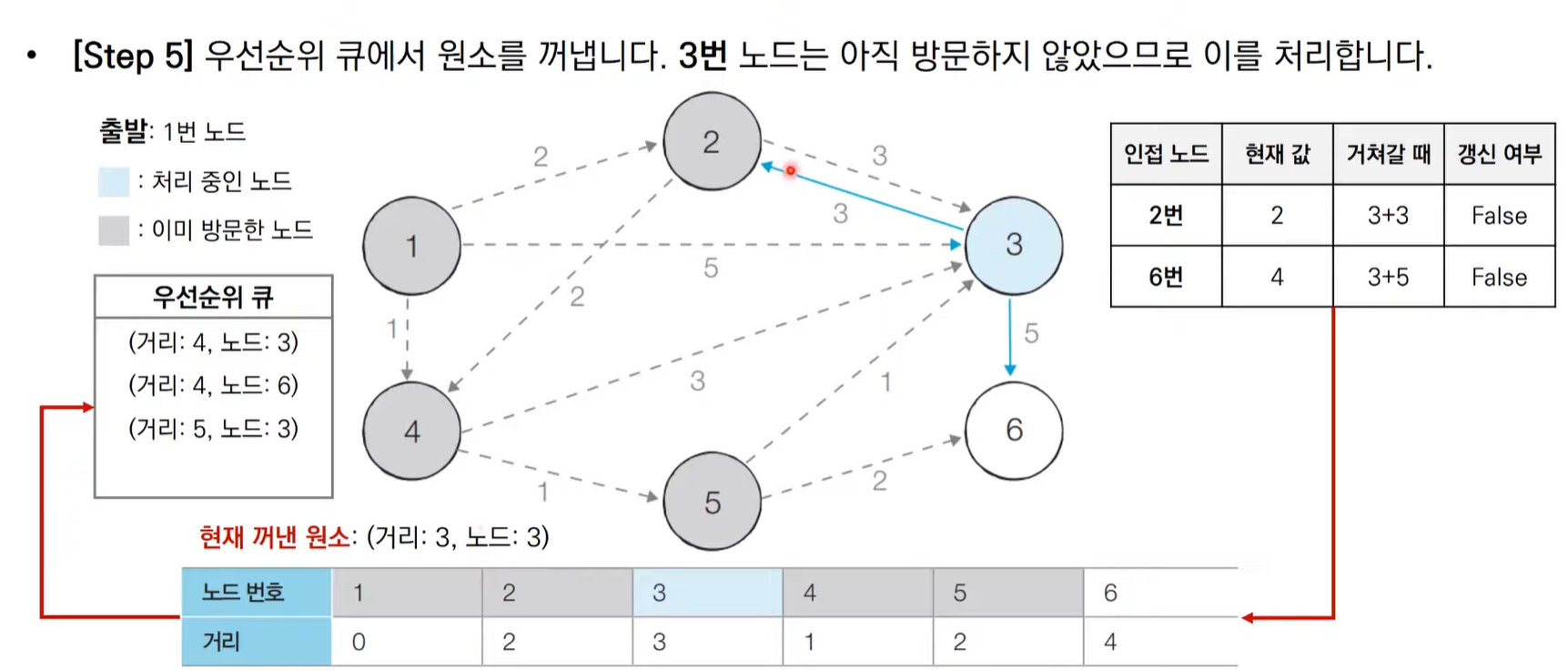
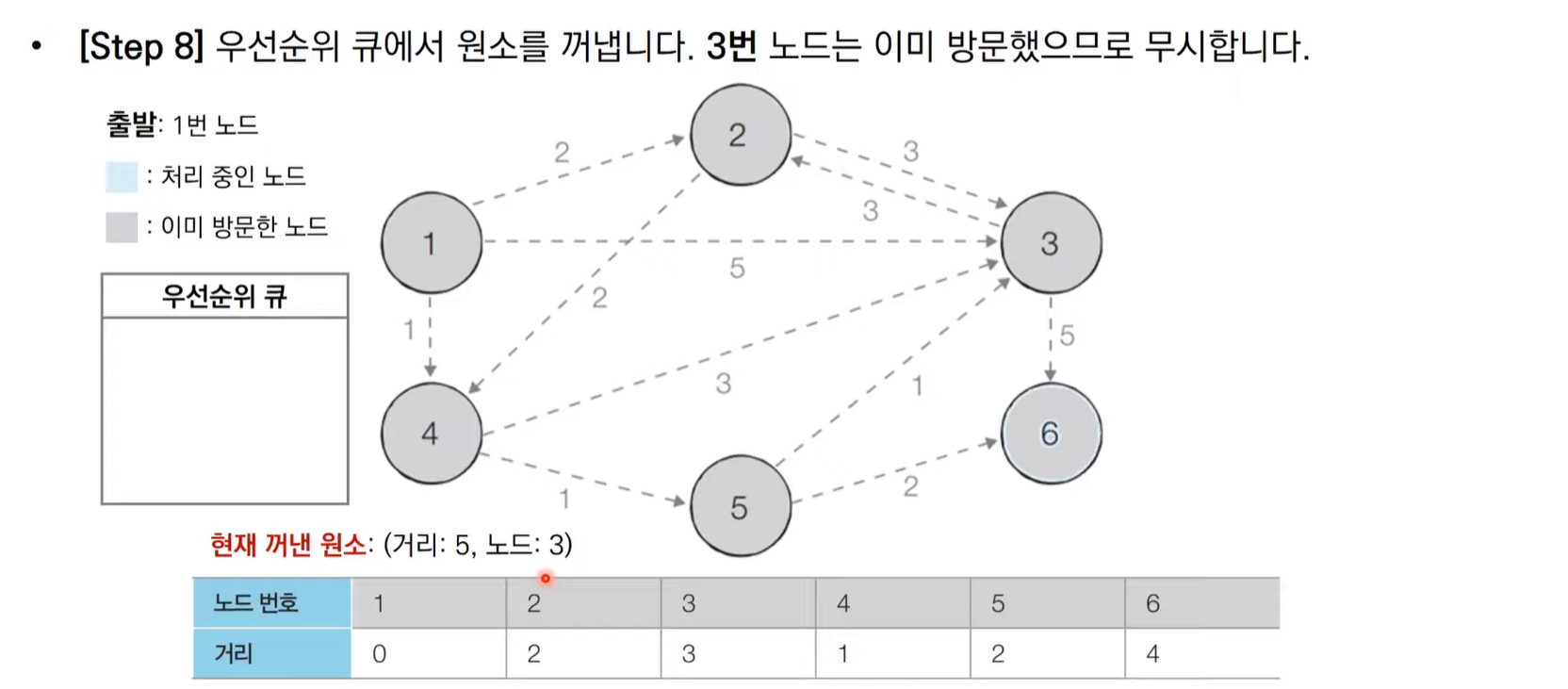
import heapq,sys
input = sys.stdin.readline
INF = int(1e9)

n,m = map(int,input().split())
start = int(input())
graph = [[] for i in range(n+1)]
distance = [INF] * (n+1)

for _ in range(m):
    a,b,c = map(int,input().split())
    # a번 노드에서 b번 노드로 가는 비용이 c라는 의미
    graph[a].append((b,c))

def dijkstra(start):
    q = []
    # 시작 노드에 대해서 초기화
    heapq.heappush(q,(0,start))
    distance[start] = 0
    
    while q:
        dist, now = heapq.heappop(q)

        if distance[now] < dist:
            continue
        for i in graph[now]:
            cost = dist + i[1]

            if cost < distance[i[0]]:
                distance[i[0]] = cost
                heapq.heappush(q,(cost,i[0]))

dijkstra(start)

for i in range(1,n+1):
    if distance[i] == INF:
        print("INFINITY")
    else:
        print(distance[i])

다익스트라 알고리즘(우선순위 큐) 성능 분석

문제 1: 전보

문제 풀이

import heapq,sys
input = sys.stdin.readline
INF = int(1e9)

def dijkstra(start):
    q = []
    # 시작 노드로 가기 위한 최단 거리는 0으로 설정하여, 큐에 삽입
    heapq.heappush(q,(0,start))
    distance[start] = 0
    
    while q:
        # 가장 최단 거리가 짧은 노드에 대한 정보 꺼내기
        dist, now = heapq.heappop(q)
        # 현재 노드가 이미 처리된 적이 있는 노드라면 무시
        if distance[now] < dist:
            continue
        # 현재 노드와 연결된 다른 인접한 노드들을 확인
        for i in graph[now]:
            cost = dist + i[1]
            # 현재 노드를 거쳐서, 다른 노드로 이동하는 거리가 더 짧은 경우
            if cost < distance[i[0]]:
                distance[i[0]] = cost
                heapq.heappush(q,(cost,i[0]))

n,m,start = map(int,input().split())
graph = [[] for _ in range(n+1)]
distance = [INF] * (n+1)

for _ in range(m):
    x,y,z = map(int,input().split())
    graph[x].append((y,z))

dijkstra(start)

cnt = 0
max_distance = 0

for d in distance:
    if d != 1e9:
        cnt += 1
        max_distance = max(max_distance,d)

print(cnt - 1,max_distance)